It may still be missing stuff from before the first person from your home instance joined the community which can make younger communities feel empty.


It may still be missing stuff from before the first person from your home instance joined the community which can make younger communities feel empty.

I’d rather have them kill shows immediately than right before the final season. See Westworld, Expanse and (almost) Snowpiercer. I’m currently really anxious about Yellowjackets.
Firefly still hurts though.

And then, Kai Patterson comes in and cuts it back down into a pretty good standard length film which I see as a win.
Nirav talks about that in the other video they released today. They’re working on it and in the meantime there is a DIY solution.
Definitely not C#. Wrong syntax for main, wrong syntax for foreach (C# has foreach (var i in someCollection) and what even is this method call syntax with =?
Edit: I dug around the website. It’s D. I’m still confused about the method call syntax though. Usually, D uses parentheses like most other C-style languages. Must be some weird syntax where you can call methods like property setters which was useful for this particular code golf challenge.
kclolck


That’s four screens total. You’re first on the chopping block.


Looks interesting but having to use it from their website feels… not great. I’d rather have something that I can still use on a train or when my router dies. Or when they decide to shut it down.
They claim that everything runs locally and doesn’t phone home. Then why do they charge 500 to 2000 bucks per month for a version that you can host on your own machine?
There seems to be an unofficial offline version but that hasn’t been maintained for years.
Have you seen how some Linux users treat people who don’t use exactly what they think is the hottest shit? Even towards people who use as much FOSS as they can but still need some proprietary stuff for certain use cases because FOSS alternatives are not quite there yet. It’s annoying as hell and comes up in every single discussion that vaguely fits the topic. I would say the comparison to Mormons is not that far off.
I’m all for moving as many people away from closed systems as possible. But tribalism and victim blaming achieves the exact opposite. Why would anyone move to something where the community makes them feel unwelcome from the start?
If we want people to use Linux, we have to be patient and help them overcome their pain points, not go “haha you dumb”.
Instead of being condescending, you might help people solve the problems that keep them from switching. Or just stay quiet. Whatever you prefer. This elitist attitude is one of the reasons why some people won’t even try Linux because they fear they will be ridiculed as soon as they need to ask for help.
Use whatever makes you happy and matches your needs. Ignore the haters.
I use arch btw. And Ubuntu. And Windows. And an iPhone. Does it matter?


I wouldn’t call it Stockholm syndrome. The problem is that even a single application that’s critical to your workflow can keep you from switching, even if everything else is much better.
I’ve switched to Linux on my laptop about 6 months ago and the overall experience is pretty good. A few annoyances that I can’t seem to fix but overall pleasant. But there are still some things that keep me from doing the same on my main workstation:
All those problems can be solved with enough patience but to be honest, I’m in my late 30s and free time is getting rare so I’d rather spend it on something that brings me joy or on learning something entirely new instead of relearning an existing skill.
And no, this not a criticism against Linux or its community. I’m just trying to give an insight into how small problems can make the switch incredibly hard, even for someone who has a degree in computer science, has worked with Linux machines for about 20 years now and would love nothing more than to leave Windows behind.


deleted by creator


Immerhin hält Appa tapfer die Stellung


DB: “At least we’re not National Rail.”
National Rail: “At least we’re not Amtrak.”
But of course, the one time, the band does something special (like play a cover of a song they’ve never done before), there is no chance of finding a video afterwards.


The emirate of Dubai a state (not a country)inside the UAE similar to how California is a state inside the USA.
Do NOT, I repeat NOT do this. Someone very close to me did something similar and got irreversible brain damage that still shows itself years later in the form of epilepsy. Our brains are not made for that little sleep over long periods of time.
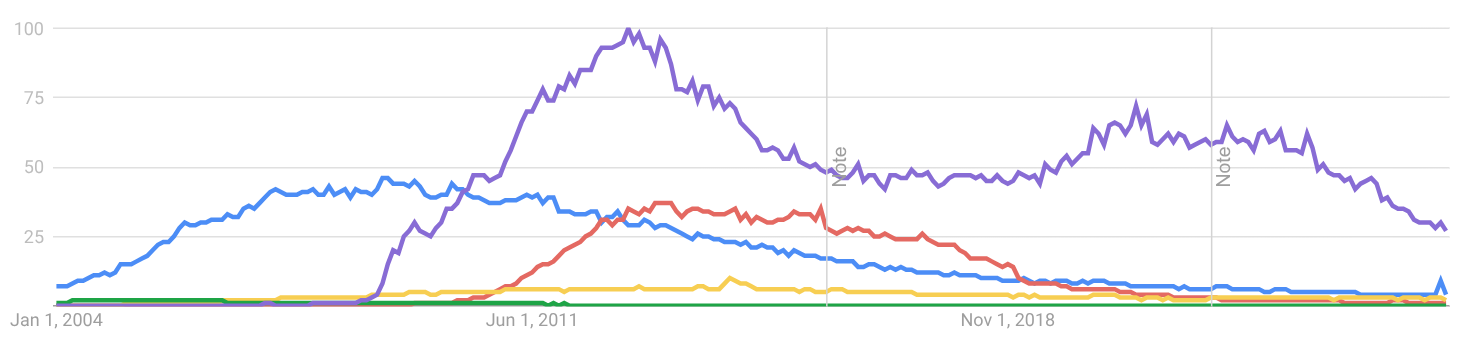
He was pretty much spot on, they came close once in August and then crossed over in October:
Interesting that Twitter isn’t on that chart even though it had been a thing for about six years at that point and had already eclipsed everything on Randall’s list in 2010